You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Grandmother, daughter and granddaughter.Riddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
That would be a daughter, mother and grandmother. 2 mothers and 2 daughters = 3 people. $7 each (3x7=21)Riddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
Three women. Two mothers. One of the mothers is the daughter of one of the mothers. This daughter is the mother of a daughter. Hence three women share $21 = $7 x 3Riddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
Grandmother, her daughter and her daughter. 21÷3 = 7 eachRiddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
$ 7 each ....1 grandmother, her daughter, & her daughter!Riddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
Three women. Two mothers. One mother is the daughter of one of the mothers. This daughter had a daughter. Hence three women $7 each = $21Riddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
Grandmother, Mother, Daughter. $7.00 eachRiddle Masters 17.03.2023
Good Morning Riddle Masters!
I've got your third and final riddle of the week!
Two mothers and two daughters go shopping. They have $21, which they split equally between them. How can this be possible?
Ooh, this is a hard one to end the week on, but we think you lot can handle it.
As always, our 'spoiler' button will hide yours and others' answers. To reveal the answers, simply click the 'spoiler' button! But do your best not to cheat.
Good luck and as always, happy riddle-ing!
Join the conversation
News, deals, games, and bargains for Aussies over 60. From everyday expenses like groceries and eating out, to electronics, fashion and travel, the club is all about helping you make your money go further.
Seniors Discount Club
The SDC searches for the best deals, discounts, and bargains for Aussies over 60. From everyday expenses like groceries and eating out, to electronics, fashion and travel, the club is all about helping you make your money go further.
Latest Articles
-
When love speaks in forgotten tongues: How dementia steals our second language

- Started by Gian T
- Replies: 0
-
Family milestone ignites debate over boundaries and attention: 'Everyone is calling her out'
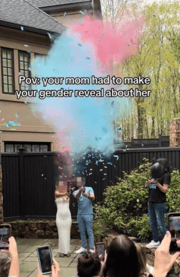
- Started by Gian T
- Replies: 0
-
SDC Tries: Woolworths’ New Ready-to-BBQ Range: ‘The Centrepiece of Convenience’

- Started by April Bradford
- Replies: 0
-
Sacred sites under siege: Urination incident highlights growing tourism tensions

- Started by Gian T
- Replies: 0
-
TV veteran's drastic move sparks vital conversation for older Australians: 'I am just disgusted'

- Started by Gian T
- Replies: 0